Written by Anssi Mirka.
“To play without passion is inexcusable.” –L. V. Beethoven
Cultivating my Mathematical Garden
I love math. It lights my day, opens my mind, brings me to bliss, gives meaning to my life like nothing else I have experienced. It challenges me like a good sport. It surprises me like a new twist in the plot of a detective story. It allows me to express myself like a poet distilling the essence of reality. I cannot get enough of it.
Our romance did not come easily, however. Definitely it was not love at first sight. Initially I treated this babe only as a tool for physics (my first love), or as a fun way to challenge myself and get cheap validation from being able to solve problems. Then I started breaking up with physics – mostly I had issues with the way it was taught – and found I enjoyed spending time more with math courses than physics courses.
That’s, of course, when I began falling for math, but at first I did it for all the wrong reasons: I was bored with physics. I felt I was better at math. The credits came easier. I could juggle more courses. I was good at puzzles. It made me feel clever… such embarrassingly immature reasons. None of them provide the healthy foundations of a strong relationship. I defined myself by the exercises solved, by the courses accomplished, by the grades gained. And the better I did, the deeper I sank into my illusion of knowledge. I thought I loved math, but in reality I was graving for acceptance. I was at the mercy of these superficial indicators, and speeding so fast I had no time to question their justification.
But the true tragedy here was this: no one—be it fellow student, lecturer, lecture material, no one—warned me about this knowledge-illusion, or clearly encouraged to look for a ‘’deeper understanding’’. If anything, I had absorbed the attitude, that a respectable mathematician should steer clear off such vague musings. In fact, I think I did not even realize there existed a higher level of mastery to aspire for. Now, I know some people trust that these profound aspects are somehow ‘’implied’’ in the standard stuff and the meanings transpire to the ‘’talented’’ ones. I don’t care to argue about the elitism of such unfinished defense, but I deeply regret to say that most of the really important insights simply do not ‘’transpire’’ even to the best of students. That is a fact. We’ll come back to this fatal fallacy after I finish my little story.
So I was speeding and about to crash, hopefully before it was too late. How did I save myself? Books. Self-study. Learning to love the math, not the scores. I was lucky, and it was not easy. This is how I re-emerged: I remember being, once again, frustrated about the course material, you know the lecture notes and the scarcity of motivation, so I sought for a good book in Amazon.com. And damn… those bibles of knowledge, those gems of wisdom, those thick, beautiful, respectable monographs. Many of them had stellar peer reviews: ’’A must-have for any aspiring analyst…’’, ‘’The best math book ever written…’’ etc. Immediately I felt I had been seriously missing out and there is a new level of skill to be reached (although at this point my idea of ‘’skill’’ was saddeningly superficial). I was thinking: ‘’Why have I been wasting my time with some last-minute put-together lecture notes when there are such dime pieces of art to be found!’’ So, I dived into literature head on—the best decision I ever made.
At first the reality hit in hard. It was impossible to keep up juggling multiple courses while studying from the fat books instead of the slim lecture notes. Not because the books were more difficult. On the contrary, as the reviews promised, they were awesome compared to most notes. But most notes are compiled together from two or three books and have conventions of their own, and that makes it two to three times more difficult to complete the course self-studying even the best book around, as compared to just cramming trough the course with the provided minimal material. As a side point, if you, like me, think we should encourage students to read literature, this is something to think about.
So, I had to give up chasing credits and courses. I could no longer draw my pathetic validation from superficial pursuits. It was either the ‘’dirty high’’ by performance or the superior leaning by books; and the right choice was painfully clear. Credit-hunting, multiple courses, and lecture notes went out the god damn door—one of the toughest decisions I ever made.
This all implied I had to build my value system anew, on the most solid ground possible: genuine love for math itself. I could afford no illusions anymore: credits were for fakers and the only thing that matters, the only thing, is math itself: how well I understand it and how beautiful I find it. There was no one to observe my process, so I had to be brutally honest about my skills. At first this was hard because of the uncertainties, self-doubts, and lack of feedback. So hard, in fact, that I really feel sorry for anyone who has to go through it alone… unless you’re a hermit, in which case it’ll be a blast. Most people, though, are not. Then again most never even learn about this option.
And so I finally learned to truly love math for its own sake. As time goes by our relationship is only becoming more interesting. Alas, it has nothing to do with being able to solve more advanced problems or collecting more theorems under my belt. No, the reason I love math more by the day is I can appreciate its beauty more purely and feel its meaning ever more strongly. Like a piano player, the more refined you become, the better you can cherish the nuances—your enjoyment has nothing, no-thing, to do with some dots on a piece of paper.
Victims of Purity
That is how I transformed from an outcome-oriented mathematician to almost purely aesthetics-oriented mathematician. I am not saying everyone should go crazy with this ‘’meta-math’’ hype, but for me it was (and is) the only way. Unfortunately, awesome as it is, it certainly hasn’t made my academic survival any easier; indeed, it would be much more efficient, in the short run, to ditch the ‘’deepeties’’ and acquire the ‘’just do it’’-attitude. I cannot do it, however. If you can, fine, but keep in mind that there at least exist other levels you should eventually aim for, if you want to excel that is. But, most importantly, never forget that there are other individuals who simply cannot survive without deeper meaning to their studies. They are precious innovators enriching our scientific idea-pool. For their sake, learn to generate motivation and provide inspiration beyond the details, the puzzles, the credits.
The reason why I am telling my story is to convince you that we must actively encourage students to seek for the beauty and beware of the temptation to ‘’just perform and pass tests’’. Do NOT make the mistake of counting on students’ ability to avoid these pitfalls on their own. It is a terrible miscalculation. The reality is that even most successful students often don’t have a clue about the true depth of the mathematical theories. This problem is so devious because it goes largely under the radar. Traditional exams and grading systems do very little to measure this dimension of learning. And the crazy thing is, that you can easily perform perfectly on those canonical examinations without cultivating any deep understanding.
How big a problem is this? Sure, some of the students simply enjoy solving puzzles and they are fine with the current system. Then there are those who are very persistent and goal driven; they might go to ‘’perform-mode’’ and become speed blind (this was me—the mindless racehorse). Usually these individuals do admirably on the traditional scales, at least up to the point when the reality kicks in. Meanwhile, however, I think most students are just withering away, coping with the curriculum. And the saddest news is this: especially the rare gems of individuals who express, at the same time, both passionate curiosity and critical thinking—the very characteristics of a scientist—are going to have it depressingly, crushingly hard. They usually—more often than not, I’m afraid—lose interest, even though they might score high on tests. This presents a huge, MASSIVE, problem for mathematics: some of our very best students become casualties of education: we’re starving our offspring, and we don’t even realize it.
Plant the Seeds
Love is never easy. First you seek for it, or at least be open to it, and then you work for it. Above all, you must learn how to share it. That is why I also love teaching math. Just like I could not enjoy life without being able to love, I cannot enjoy math without being able to share it, teach it.
And we need to teach the students to love math for its own sake. If we only teach technical skills we drive away the most passionate individuals, and those that survive are doomed to remain disabled to communicate math with radiating love. I could not care much less about the technical skillset, neither my own nor my students. That is not the essence of mathematics, not the true form of understanding. Yes, one must know how to play an instrument a bit before delivering a musical masterpiece; or know how to read before opening a book on poems, but if you want to inspire greatness, you must provide the experience of joy. You must plant the seed of knowledge: wonder.
It is, therefore, not enough to present the proofs and details to the students and hope that they somehow know how to dig into the deeper message; that they automatically draw passion out of the deltas and epsilons. My experience with students of all skill levels tells me it just won’t work like that: the development of passion and intuition requires, initially at least, a clear view from the right vantage point and a good injection of passionate interpretation. Each student, of course, is different and ideally one should leave room for an individual discovery, but if you only hand out the details you’re leaving it to the chance—no, you’re fooling yourself and letting your students down. I’ve said it before, I say it again: Every passing day promising students are unnecessarily losing their interest and bitterly giving up math. And for no better reason that no-one has ever showed them how things could be, lifted off the veil of obscuring details, inspired them with fulfilling joy. Finally, again and again it seems to be the case that the more passionate you already are, the more difficult it becomes to survive here. The situation is maddeningly messed up. Even if one manages to develop a hunger for the divine food that is the beauty of mathematics, one receives no help in gathering it; the very first step in the path to passion is loaded with hardships. Eventually, then, one either withers away or, maybe even worse, forgets the hunger and settles for coping with unnourishing food.





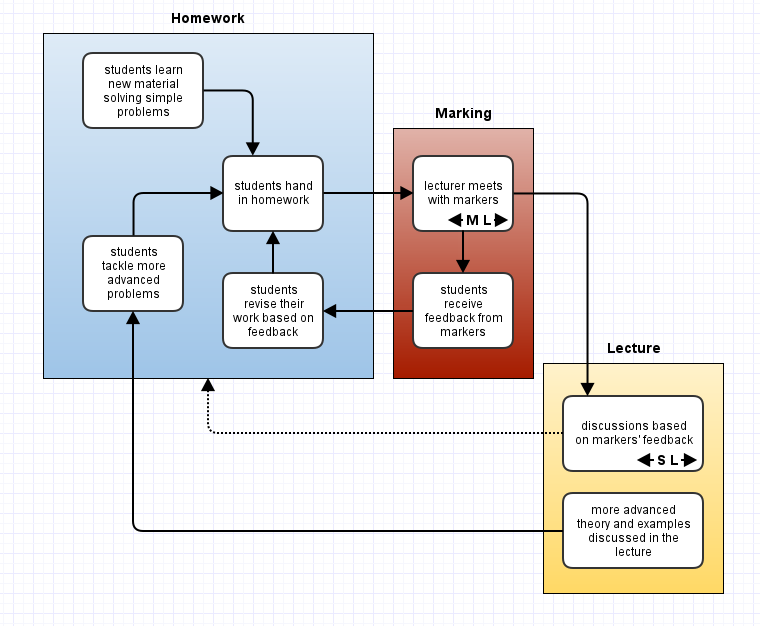
 This is how we came up with the idea of a proof hat. It makes it possible to distinguish between different kinds of justifications. When teacher writes a formal justification for a claim, he/she wears the proof hat. When a topic is discussed more informally, the proof hat is not used.
This is how we came up with the idea of a proof hat. It makes it possible to distinguish between different kinds of justifications. When teacher writes a formal justification for a claim, he/she wears the proof hat. When a topic is discussed more informally, the proof hat is not used.