Kokemuksia uudesta arviointitavasta matematiikan yliopistokurssilla

12.6.2019 | Jokke I A Häsä

”Tämä oli minulle normaalia matematiikan suorittamista huomattavasti motivoivampaa”

Kurssille Johdatus yliopistomatematiikkaan suunniteltiin keväällä 2018 kokonaan uusi arviointitapa. Kyseessä on matemaattisten tieteiden kandiohjelman ensimmäisen syksyn tai kevään 5 opintopisteen kurssi, jolla opitaan matemaattista ajattelua ja viestintää sekä perusasioita joukoista, kuvauksista ja muista tutkimusmatematiikan peruskäsitteistä. Kurssilla oli ollut käytössä väli- ja loppukoe, joihin sai lisäpisteitä laskuharjoituksista. Olimme uuden luennoitsijan, Mikko Stenlundin kanssa kuitenkin sitä mieltä, että tenttiarviointi ei oikein sovellu tälle kurssille. Tenttiarviointi nimittäin ohjaa opiskelijoita työskentelemään loppupainotteisesti ja pänttäämään ”tärppejä”, jotka helposti unohtuvat tentin jälkeen.

Kurssi on suurimmalle osalle opiskelijoita ensimmäinen matematiikan kurssi. Siksi halusimme alusta alkaen motivoida opiskelijat säännölliseen viikoittaiseen työskentelyyn. Toisaalta kurssilla opitaan asioita lähinnä tulevaa matematiikan opiskelua varten, joten emme halunneet, että opiskelijat opiskelevat asiat tenttiä varten ja unohtavat ne heti sen jälkeen. Lisäksi kurssille haluttiin lisätä itse- ja vertaisarviointia, koska nämä tukevat tutkimusten mukaan opiskelijoiden pystyvyyden kokemusta, hyviä opiskelustrategioita ja elinikäistä oppimista.

Kurssilla on hyvin heterogeeninen osallistujajoukko. Alla olevassa kaaviossa näkyy syksyn 2018 kurssin suorittaneiden jakauma eri koulutusohjelmiin. (Vanhoissa koulutusohjelmissa opiskelevat on kaaviossa pyritty yhdistämään vastaavaan uuteen koulutusohjelmaan.)

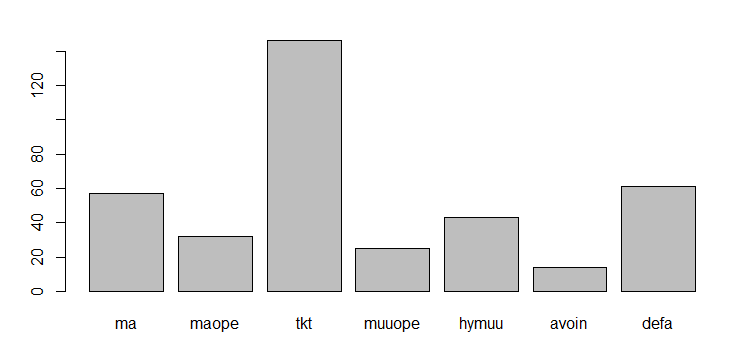
Kaavio suorittaneista eri koulutusohjelmissa

Syksyllä 2018 kurssin suorittaneiden jako eri koulutusohjelmiin (ma = matemaattisten tieteiden kandiohjelma, maope = matematiikan, fysiikan ja kemian opettajan kandiohjelma, tkt = tietojenkäsittelytieteen kandiohjelma, muuope = muu Helsingin yliopiston opettajaohjelma, hymuu = muu Helsingin yliopiston tutkinto-ohjelma, avoin = Avoimen yliopiston kautta suorittava, defa = Digital Education For All -hankkeen kautta suorittava)

Suunnittelimme Mikon kanssa uuden arvioinnin, jossa ei ole lainkaan tenttiä, vaan arvosana perustuu suoraan erilaisista tehtävistä saataviin pisteisiin. (Arvioinnin toteuttamiseen osallistui myös kurssin toinen luennoitsija Timo Hänninen.) Kurssilla on käytössä Tehostetun kisällioppimisen menetelmä, jossa uusia asioita opiskellaan tehtävien avulla. Osa tehtävistä arvioidaan opettajien toimesta tai automaattisesti, ja osassa tehtäviä pisteitä saa pelkästä yrittämisestä.

Oheisessa taulukossa näkyy erilaisten tehtävien painotus arvosanassa. Viikkotehtävillä on hyvin suuri painoarvo. Suurin osa pisteistä tulee tehtävän laadun perusteella, mutta myös pelkällä tekemisellä on merkittävä rooli. Laadun perusteella arvioitavista tehtävistä saa palautetta joko opettajalta tai tietokoneelta, ja näitä saa palautteen jälkeen korjata ja palauttaa uudestaan.

Tehtävätyyppi	suorituksen laatu	yrittäminen
Viikoittaiset harjoitustehtävät	45 %	25 %
Itsearviointiharjoitukset	–	10 %
Kurssin lopun laaja tehtävä	15 %	–
Puolenvälin laajan tehtävän vertaisarviointi	5 %	–

Kokonaan uutta kurssilla oli projektityyppinen lopputyö, joka nimettiin ”laajaksi harjoitustehtäväksi” kurssilla Algebra II tehtävän vastaavan työn mukaan. Lopputyössä opiskelijoille annetaan osaksi tai kokonaan uusi matemaattinen käsite, johon heidän on tutustuttava. Työssä opiskelijoita pyydetään muun muassa laatimaan uudelle käsitteelle matemaattinen määritelmä sekä lukemaan ja kirjoittamaan yksinkertaisia todistuksia käsitteeseen liittyen. Käsitettä on tyypillisesti myös kuvailtava omin sanoin ja piirroksin. Lopputyötä harjoitellaan kurssin puolessa välissä. Lopputyöstä saa ennen lopullista palautusta vertaispalautetta toisilta kurssilaisilta, ja lopullisen version tarkistavat ja pisteyttävät kurssin opettajat.

Syksyllä 2018 kerätyssä kurssipalautteessa kysyttiin uuteen arviointimenetelmään liittyviä kysymyksiä. Oheisesta taulukosta ja kaaviosta näkyy opiskelijoiden vastausten jakauma Likert 1–7 -asteikkoisiin kysymyksiin (1 = olen täysin eri mieltä, 7 = olen täysin samaa mieltä; n = 286). Erityisesti olimme iloisia, että opiskelijat kokivat vahvasti voivansa hyödyntää oppimaansa tulevilla kursseilla ja että he haluaisivat osallistua samalla tavalla suoritettavalle kurssille uudestaan.

Kysymys	keskiarvo	keskihajonta
Pystyin näyttämään osaamiseni kurssilla ja sain siitä tunnustusta	4,92	1,45
Koen voivani hyödyntää oppimiani asioita tulevilla kursseilla	5,69	1,37
Haluaisin osallistua tällä tavalla suoritettavalle kurssille uudestaan	5,73	1,50

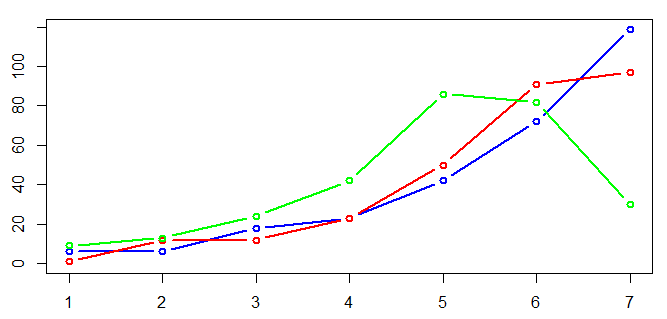
Likert-vastauksia kurssilla JYM, syksy 2018

Vastausten lukumääriä Likert-asteikkoisiin palautekysymyksiin (vihreä = ”Pystyin näyttämään osaamiseni ja sain siitä tunnustusta”, punainen = ”Koen voivani hyödyntää oppimiani asioita muilla kursseilla”, sininen = ”Haluaisin osallistua tällä tavalla suoritettavalle kurssille uudestaan”)

Numeeristen vastausten lisäksi opiskelijat vastasivat avoimeen kysymykseen ”Mitä mieltä olet kurssin suoritusmenetelmästä?” Vastaukset olivat lähes poikkeuksetta positiivisia: 286 vastauksen joukossa oli vain seitsemän kriittistä vastausta (2,4%).

Vastauksia analysoitiin aineistolähtöisellä laadullisella sisällönanalyysillä, jotta saataisiin selville, mitä hyötyä opiskelijat kokevat saavansa uudesta arviointimenetelmästä. Vastaukset voitiin ryhmitellä kolmeen kategoriaan.

Suurimmassa kategoriassa opiskelijat korostivat, että arviointimenetelmä motivoi työskentelemään jatkuvasti koko kurssin ajan. He sanoivat tällaisen jatkuvan työskentelyn tukevan heidän oppimistaan, ja lisäksi tukevan hyvää työskentelyrutiinia.
Toiseksi suurimmassa kategoriassa kehuttiin kurssin kokeettomuutta. Vastaajien mukaan se vähentää stressiä ja kannustaa keskittymään pänttäämisen sijaan asioiden opiskeluun ja omaan oppimiseen.
Kolmannessa kategoriassa viitattiin siihen, että pisteiden jatkuva kertyminen antaa paremman kuvan osaamisesta kuin yksittäinen tentti.

Ohessa joitakin kuvaavia sitaatteja opiskelijoiden vastauksista.

”Pidin siitä, että kurssi kannusti tekemään tehtäviä jatkuvasti, jolloin oppiminen oli palkitsevampaa ja paremmin mieleen jäävää, kuin jos esimerkiksi kurssi suoritettaisiin yhdellä tai useammalla kurssikokeella, joihin pitäisi valmistautua erikseen.”

”Tehtäviin eikä tenttiin painottava arviointi kannustaa opiskelemaan itseä, ei tenttiä varten.”

”Tämä oli minulle normaalia matematiikan suorittamista huomattavasti motivoivampaa. Aikasemmat kokemukseni ovat lukio- ja amk-matikasta, missä oksennetaan useiden kuukausien työ yhdelle tenttipaperille […] Aiemmin on ollut hirveä kammo tätä oppiainetta kohtaan, ja lähdinkin mukaan haastamaan itseäni. Yllätys oli iso kun tajusin, että konkreettisesti kaikki työ mitä jaksan tehtäviin laittaa, näkyy kurssin loppuarviossa.”

Joissakin palautteissa esiintyi kuitenkin myös kritiikkiä kokeettomuutta kohtaan:

”Minusta tässä uudessa systeemissä että loppukoetta ei ole ja kurssinumero tulee vain sen perusteella miten hyvin suoriutuu laskuharjoituksista […] on se ongelma että tässä arvosana kertoo sen miten hyvin asian on osannut silloin kun sitä on vasta opetellut, eikä sitä miten hyvin asian osaa kurssin jälkeen […] Tämän takia loppukoe antaisi minusta paremman arvion siitä miten hyvin opiskelija osaa kurssin asiat.”

Yleisesti ottaen opiskelijapalautteessa esiintyi sekä kehuja että kritiikkiä, joka otetaankin huomioon seuraavissa toteutuksissa. Esimerkiksi tehtäviä koettiin olevan liian paljon opintopistemäärään nähden, ja osa opiskelijoista koki laajat harjoitustehtävät ahdistaviksi, koska ne olivat niin avoimia ja soveltavia. Tehtävämäärää pyritään jatkossa vähentämään, ja laajoja harjoitustehtäviä ohjeistamaan paremmin, jotta ne eivät säikäytä opiskelijoita.

Positiivisena huomiona on lopuksi todettava, että palautteen mukaan kurssi on lisännyt monien opiskelijoiden kiinnostusta matematiikkaa kohtaan. Osa opiskelijoista on sanonut haluavansa kurssin myötä ottaa lisää matematiikan kursseja tai ottavansa matematiikan sivu- tai jopa pääaineeksi. Kaiken kaikkiaan kurssia ja sen suoritusmenetelmää on pidettävä onnistuneena, ja sen kehittämiseen edelleen kannattaa panostaa!

”Kiitoksia erinomaisesta kurssista! Kurssi herätti itselläni syvällisemmän mielenkiinnon matematiikkaa kohtaan, jossa aiemmin en ole kokenut olevani erityisen hyvä. Tämä toimi mielestäni hyvänä esimerkkinä miksi valita Helsingin Yliopisto kaikista vaihtoehdoista. Toivottavasti vastaavia kurssimuotoja on saatavilla aineopinnoissakin!”

Tässä kirjoituksessa esitetyt uutta arviointimenetelmää koskevat tulokset esiteltiin ensimmäistä kertaa Pedaforum 2019 -konferenssissa Kumpulan kampuksella 6.6.2019.

Greetings from CERME11 conference

7.3.2019 | Jokke I A Häsä

By Jokke Häsä and Juuso Nieminen

Preparing for the opening ceremony in the Domkerk

In the beginning of February, the 11th Congress of the European Society for Research in Mathematics Education (CERME11) was held in Utrecht, Holland. There were two representatives from our department, myself and Juuso Nieminen, and a few more from the Department of Education.

It was quite a surprise to find that the main conference venue was St. Martin’s Cathedral! As grandiose as the premises were, it was rather cold inside and from time to time it was difficult to hear the keynote speakers because of the echo. However, it was an amazing experience, and fortunately all the small group presentations were held in different locations throughout the beautiful small city.

CERME has a very inclusive reputation, and there were around 900 participants from all fields of mathematics education. The conference has been expanding rapidly during the last couple of years. The participants were divided into so-called Thematic Working Groups (TWGs), and everyone stayed with their group throughout the conference.

The pub cat at Oudaen restaurant and brewery

Juuso and I gave presentations in different TWGs. The theme in my group was assessment. We discussed, for example, the growing role of automatic assessment, validity questions of national examinations and the need for more articulated theoretical frameworks for formative assessment in mathematics. Juuso was in the diversity group, where mathematics education was placed in the wider context of culture, society and the political. The TWG especially addressed teaching mathematics to a diversity of students, taking into account the social and cultural aspects.

Conference dinner was held in the Railway Museum

My presentation (written together with Johanna Rämö and Viivi Virtanen) dealt with validity issues concerning the new digital self-assessment model (DISA) used, for example, in our linear algebra courses. Juuso talked about Universal Design in the context of mathematics; it is a framework for building learning environments to address the needs of student diversity. This frame is often used in relation to learning disabilities. Both papers will appear in the conference proceedings shortly.

The conference paraded a very enjoyable and inclusive collegial atmosphere. It was a huge pleasure to meet old colleagues and make new acquaintances, discussing everything about mathematics education from policy making and cultural impacts to everyday classroom activities and decisions. And all in the wonderful city of Utrecht, by the cathedral and canals and the slowly emerging spring.

: Leiden tour

: Castle tour

The Durham Experience, Part II

18.12.2015 | Jokke I A Häsä

In the academic year 2014–2015 I had the privilege to teach a lecture course in Durham University in the United Kingdom. Durham is a rather prestigious university that is very selective in its student intake. In these entries I record some of my thoughts concerning the experience. Although I could only witness the system in Durham, many details can most likely be generalised to other UK universities.

(Read the previous post here.)

Lecturing Algebra II

Despite its name, Algebra II is the first course in abstract algebra offered in Durham. It is a second-year course, spanning the whole academic year. Compared to courses taught for example in Helsinki, the course covers roughly the material for a basic one-term algebra course plus a slightly shorter and more advanced course. The course had about 170 students.

I was eager to face the challenge of teaching a full-fledged lecture course in a new country. Having taught algebra before, I knew it to be a difficult topic that demands a lot of abstract thinking and becoming familiar with many new concepts. My colleagues in Durham had also warned me that it would be very difficult to get the students to appreciate how much and what kind of work they would have to do in order to learn the material effectively.

While I was planning my course, it soon became clear to me that to encourage the students to work in an effective manner for their own learning, I should implement methods that in the University of Helsinki go under the name “Extreme Apprenticeship Model”. This model has been applied to teaching certain mathematics undergraduate courses in the Department of Mathematics and Statistics since 2011. After its introduction, the model has enjoyed great success. In particular, it has been successful in engaging the students, teaching them good, professional-style studying habits, as well as making them more committed to their studies in the department.

It was, however, impossible for me to run a full-fledged Extreme Apprenticeship course since I had much less control over the teaching resources than is usual in Finland. Therefore I needed to adapt my teaching to a new environment. In such cases I find it useful to consider the motivations behind the practices in order to decide what is essential and what is not. Doing so, I found that I wanted to focus on

Improving the students’ working habits.
Enabling strong bi-directional feedback that would allow the students to know what is expected from them and the teaching to be guided by the students’ needs.
Teaching abstract thinking and communicating these thoughts in speaking and writing.

Considering these points led me to implement roughly the following set of methods:

a form of flipped learning, in which the students would work individually for their own learning, and the lectures are used to solidify the students’ knowledge, build a general picture, weed out misconceptions, and discuss effective study methods
course notes designed for the students to be able to read and understand to a great extent by themselves
detailed descriptions of learning objectives and self-evaluation exercises based on them
weekly meetings with homework markers to ensure they would know what I require of the students, and that I would find out how the students are doing
the possibility of resubmitting certain homework questions in order to employ the markers’ comments if the first solution was not good enough
activating the students during the lectures with small group tasks, questions, and polling exercises
activating conceptual thinking through tasks that would require explaining, connecting and evaluating.

In short, the course ran in the following way. First, the students were given typed, well-prepared notes. They familiarised themselves with new concepts through simple homework problems. They would hand in the problems to be marked. Once the markers had had a few days to look at the scripts, we would meet together to discuss what the students had come up with. Then the markers would go back to finish the marking, while I would discuss the most common mistakes and important misconceptions in the lectures. I would use online voting systems in the lectures to help gauge the students’ learning pace and to promote meaningful discussions. The lectures would also progress to deeper theory and examples, and the next week’s homework would – in addition to preparatory material – also contain harder problems on topics that were discussed during the week.

Running Algebra II. The arrows between boxes of different colour indicate the flow of information or feedback. The double arrows ML and SL symbolise bi-directional feedback between markers and lecturer and between students and lecturer.

There were two types of questions in the homework. Most of the questions were marked based on honest attempt whether the answer was right or wrong. For these questions, the markers were not required to comment anything if they did not have time for it. The students could later compare their solutions to the model solutions. On the other hand, a few questions were more substantial, usually requiring a written proof. These questions were marked based on the quality of the answer – not only of the mathematical correctness, but also the quality of writing. It the solution was not adequate in the first week, the student was encouraged to resubmit the question the following week and would receive the marks after the resubmission.

In applying the above methods, I faced several challenges. As already mentioned in my previous post, the lecture system in Durham is very rigid. My lecturing hours (twice 50 minutes a week plus a fortnightly “problems class”), as well as tutorials (50 minutes per a 15 person group fortnightly) were all prescribed to me. Also the teaching assistants giving the tutorials and marking the homework (mostly separate people, to my surprise) were decided for me. One major difference with Extreme Apprenticeship method in Helsinki was that I could not provide the students with a drop-in workshop with instructors that would always be present. Another challenge was that the other teachers in Durham that I got into contact with had very little experience about any of the methods I was going to apply, so I could not count on their advice as much as I was used to in Helsinki.

The biggest obstacle, however, was that the students were in general unaccustomed to anything else than the traditional teaching style where the lecturer writes on the blackboard and students take notes, which they then later refer back to when doing their homework. This limited background, of which I only learned gradually when I was already teaching the course, created a lot of mental opposition towards my teaching right from the start of the course. Of course, I was prepared to explain any new methods I would be using, so that the students would be aware of what was expected from them and how they would benefit from the diverse exercises, but in Durham I felt I was required to explain myself much more than usual. There was a constant need to reassure the students of the fact that they were indeed learning the necessary skills even when they did not recognise the “unconventional” methods we were using.

Despite initial misgivings, the students gradually learned to work in the new way, some quicker, some at a slower pace. However, student feedback questionnaires half-way through and at the end of the course show that the students were not in general happy with the lectures, saying that these were useless and not teaching them anything. Being inefficient is a well-known problem of mass lectures, and the amount of negative feedback on this aspect seems only to indicate that the students have not previously recognised this inefficiency. On the other hand, the prepared course notes received a lot of positive feedback. It seems to be very rare in Durham that the students would be given such complete sets of notes.

During the course, I observed the students closely in order to spot any learning difficulties resulting from the change in teaching style. Some individuals indeed claimed that they were facing serious difficulties, but on the whole I could see no significant indication that anything would be going wrong. Even if the students complained, they were still clearly learning. Their results in the final exam were as good as would be expected from such a course in general.

While one might not experience any obvious adverse effects from the different kind of teaching, it would be good to know whether my efforts actually made a difference in the end. From the teacher’s point of view, I certainly felt that I was spending my time much more meaningfully than I would have using a more traditional style, and that kept me highly motivated throughout the year. Many students also changed their minds about my methods and gave me praise in private emails and conversations. One student said that I had taught him to write proofs, and claimed that this had helped him in the exams also on other courses. Some physics students thanked me for giving examples of physical applications and for teaching not only the content but also the method of studying mathematics. Another student, who had originally despised my lecturing style, thanked me in the end, saying that during the course he had realised the value of his own work and that this was the most important thing he had learned from the course.

The last mentioned comments show that the methods I employed were about so much more than straightforward delivery of definitions and theorems. The students were taught to read and write mathematics on their own and construct their personal understanding instead of simply memorising results and methods. Although this kind of instruction may be unusual to see in the Durham maths community, I trust that my example shows that, if found valuable, it is fully possible to implement.

The Durham Experience, Part I

23.7.2015 | Jokke I A Häsä

Durham undergraduate system

Compared with Finland, the English universities hold a much tighter grip of both students and the teaching staff. For someone used to making all the decisions themselves, from planning your own schedule as a student to choosing the most suitable date for your exam as a lecturer, the inflexibility of the UK system may come as a shock. On the other hand, there is also a feeling of security and comfort when things are planned for you in advance.

Studying an undergraduate degree in mathematics in England takes typically 3–4 years and costs up to 9000 pounds in tuition fees per year (Scotland has no tuition fees). As a maths student, you will not be studying any other subject, although courses in some subjects such as theoretical physics can in manyuniversities be incorporated in a maths curriculum. You will be able to choose between a few optional courses, but mostly the schedule will be decided for you. You will simply receive a timetable in the beginning of each year telling you what lectures you will be taking and when.

In Durham, a typical maths course (they are called “modules”) takes a full academic year, consisting of lectures, tutorials and homework assignments. There are three terms: autumn, spring and summer (these are dubbed “Michaelmas”, “Epiphany” and “Easter”, respectively). To give a sense of how the three terms are organised, this year the autumn term lasted from 6 October until 12 December, spring term from 12 January until 13 March and summer term from 20 April until 19 June. Spring term ends at Easter, after which there is a five-week holiday before the start of the summer term. The summer term is aimed at revision: there are 2–3 weeks of revision classes, and then the exams begin.

All exams are held in the summer term, in either May or June, regardless of when the course itself took place. On a typical course, the final grade depends entirely on the exam. Homework or mid-term exams, if any, will not contribute. If a student fails in the exam, they can retake it in August, but in the resit it’s not possible to score more points than the minimum for a pass. No further resits are allowed.

After the exams, the students wait in anguish until all the scripts have been graded before they receive their final grades. They are not allowed to view their own scripts, at least not in the maths department, nor even know which questions scored them points and which did not. This is in sharp contrast to the practice for example in the maths department in Helsinki, where the exam, its solutions and some notes on the grading are published, together with question-by-question statistics, and where a student can at any time request to see their script in presence of the lecturer.

Let us switch to the teacher’s point of view. As for the students, the timetable is completely decided in advance, and the teacher cannot affect the amount or length of lectures (typically two 50-minute lectures a week in Durham) or tutorials (50 minutes per a 15 person group fortnightly). The content of the course is also prescribed, but in practice you are allowed to take some liberties.

The final exam, as well as the resit exam, need to be written in January, which can be quite difficult as half of the course is yet to be lectured. (Even more so for those teachers who are teaching a half-year course starting in January!) After the exam is ready, together with solutions and a grading scheme, it will be checked by a colleague, and subsequently by an external checker from another university. This is to ensure the exam is of a suitable level of difficulty and free of errors. After the exam, there is a fervent period of grading, which has to be done in a week or so for a typical exam. The grading is also checked by a colleague for omissions or mistakes in adding the points.

For a Finn, all this railroading seems a bit excessive. Not being able to retake the exam more than once, to retake it for a better grade or to manage one’s timetable oneself seems to make the students’ life rather stressful. There is a tendency to work less in the autumn and then attempt to absorb most of the content in the revision period. The stress and worry of the students is also reflected in the course feedback: if the students feel the teacher is not preparing them well enough for the exam, their criticism often worded in strikingly harsh language.

However, the students are not left to struggle on their own, but actually rather conscientiously taken care of. Each student is assigned an “academic advisor”, a permanent staff member with whom the student has regular meetings throughout the year. Each student’s homework activity and tutorial participation is monitored, and if there is cause for concern, the student is contacted for an explanation. This manages to ensure that the students keep doing their homework even though the homework does not count towards their final grade.

The staff enjoys similar fostering. Every new lecturer gets a “mentor” who can be approached if case of unforeseen problems, practical or otherwise. The mentor will also observe the teaching of their mentee to see that everything is going well. Furthermore, every teacher is asked to observe at least one lecture or tutorial of another randomly selected teacher. I find this system quite appealing, as following someone else’s class very often gives me new ideas and revelations. I also found the checking of other people’s exams very useful. One can quickly become blind to one’s own mistakes, and it is easy to misjudge the skills of the students, especially in a new environment. My own exam was much improved after hearing the comments of my checker.

Another detail in the Durham system I was happy about was the three-week revision period and the holiday preceding it. During that time I felt that the pieces were finally coming together in the students’ minds. I have had a similar experience during my own time as a student, when for some reason I have not taken the exam at the first possible instance, but rather deferred it a couple of weeks. It made me much more confident in the actual situation, and I’m sure the same is true with many of my Durham students. If only a similar revision period could be arranged without having to delay all exams until the end of the year!

In conclusion, the system of undergraduate studies in the UK seems quite strange from a Finnish point of view, and I cannot say I appreciate the feeling of lack of freedom. I’m afraid the majority of students will have difficulties growing to be independent thinkers when they have so little to say regarding their own studies. Also the teachers have to strive really hard if they want to experiment and develop their own teaching into a new direction. However, there is definitely something to learn about in the way students and lecturers are tended and their progress constantly followed. To the students such instruction is certainly very helpful, as they are often not yet as independent as we might think them to be.

Aktiivisempia luentoja

2.6.2014 | Jokke I A Häsä

Kirjoittaneet Jokke Häsä ja Johanna Rämö.

Miten opiskelijat saisi pidettyä hereillä luennoilla? Mistä tietää, pysyvätkö kuulijat kärryillä?

Olemme yrittäneet ratkoa näitä ongelmia muun muassa kokeilemalla erilaisia reaaliaikaisia luentopalautejärjestelmiä. Perinteisesti reaaliaikainen luentopalaute on toteutettu ns. klikkereillä, pienillä kaukosäätimen tapaisilla laitteilla, jotka jaetaan opiskelijoille ja joiden avulla he voivat äänestää oman vastauksensa luennoitsijan asettamiin kysymyksiin. Klikkereitä voi käyttää sekä testaamaan opiskelijoiden osaamistasoa että keräämään palautetta luennon edistymisestä.

Klikkereitä on kuitenkin harvoin tarjolla kaikille erityisesti suurilla massaluennoilla. Nykyisin on tarjolla erilaisia nettipohjaisia äänestysjärjestelmiä, joita on lueteltu muun muassa Aalto-yliopiston VipuPiste-blogissa. Opiskelijat voivat äänestää älypuhelimilla tai kannettavilla, ja opettaja saa tulokset netin välityksellä omalle koneelleen. Kaikilla ei välttämättä ole tarvittavaa laitetta mukanaan, mutta yleensä laitteita on yhteensä salissa niin paljon, että opiskelijat voi pyytää vastaamaan 2-4 hengen ryhmissä.

Kokemuksemme mukaan luennoitsijan ei kannata pitää opiskelijoiden äänestystuloksia omana tietonaan, vaan heijastaa ne kootusti kaikkien nähtäville. Tällä tavoin opiskelijat säilyttävät itsekin tuntuman koko ryhmän tasosta. Useinhan on niin, että yksittäinen opiskelija ei uskalla esittää “tyhmää kysymystään” kaikkien kuullen, vaikka tosiasiassa sama kysymys on mielessä useammallakin, joskus jopa suurimmalla osalla. Äänestystulosten näkeminen – varsinkin jos moni on erehtynyt oikeasta vastauksesta – saattaa tällä tavoin madaltaa opiskelijan kynnystä esittää omia kysymyksiä.

Luentoäänestyksiä voi käyttää eri tarkoituksiin:

Keskustelun herättämiseksi
Yleisen harhakäsityksen esiin tuomiseksi
Opiskelijoiden oman ajattelun aktivoimiseksi
Rytminvaihdokseksi pitkälle luennolle

Yleensä äänestykset tukevat useita näistä tavoitteista yhtäaikaisesti. Palautejärjestelmiä on myös käytetty järjestelmällisesti jonkin tietyn opetusmenetelmän toteuttamiseen. Jyväskylän yliopiston fysiikan laitoksella Pekka Koskinen on käyttänyt luentoäänestyksiä toteuttaakseen Eric Mazurin Peer Instruction -tyyppistä opetusmenetelmää (Koskinen esitteli kokemuksiaan Arkhimedes-lehden numerossa 3/2012). Peer Instruction -metodissa opiskelijat vastaavat ensin luennoitsijan esittämään kysymykseen ja heille näytetään äänestyksen tulos. Sen jälkeen heidän annetaan keskustella toistensa kanssa ja äänestää uudestaan. Tällä tavoin opiskelijat saadaan itse korjaamaan omia alkuperäisiä käsityksiään vuorovaikutuksessa toisten kanssa

Luentopalautejärjestelmiä on myös mahdollista käyttää opiskelijoiden kysymysten ja kommenttien keräämiseen. Palautekanavan voi jättää auki luennon ajaksi, jolloin opiskelijat pystyvät sen kautta kysymään luennolla heräävät kysymyksensä.

Suurin haaste luentoäänestyksissä on hyvien kysymysten keksiminen. (Tuntuu siltä, että matematiikassa tämä on jotenkin erityisen hankalaa.) Oikea vastaus ei saa olla ilmiselvä, sillä muuten äänestämisessä ei ole mieltä. Monesti parhaat kysymykset ovat sellaisia, joihin ei edes ole yhtä ja ainoaa oikeaa vastausta. Silloin niistä syntyy mielenkiintoisia keskusteluja. Myös harkittu epämääräisyys kysymyksenasettelussa voi olla hyödyksi.

Alla on joitakin esimerkkejä käyttämistämme luentokysymyksistä. Kaksi ensimmäistä ovat perinteisempiä kysymyksiä, joista ensimmäisen on tarkoitus treenata kuvaajan tulkintaa ja jälkimmäisen lineaarialgebrassa esiintyvän “vapauden” käsitettä. Kumpikaan kysymys ei kuitenkaan ole aivan suoraviivainen, vaan opiskelijan on syvennyttävä kuhunkin vastausvaihtoehtoon.
Seuraavat esimerkit ovat soveltavampia. Ensimmäisessä opiskelijan on mietittävä, mitä ominaisuuksia on hyvällä matemaattisella määritelmällä. Yhtä ainoaa oikeaa vastausta ei ole, vaan vastausvaihtoehdoista voidaan keskustella.Viimeisessä esimerkissä annetaan opiskelijan assosioida vapaasti.
Kurssipalautteessa opiskelijoiden suhtautuminen on ollut enimmäkseen myönteistä. Esimerkiksi Avoimen yliopiston lineaarialgebran kurssilla kysymykseen “Kuinka hyvin luentokysymykset tukivat oppimista?” keskiarvo 25 vastanneen kesken asteikolla 1-5 oli 3,84. Vapaista kommenteista suurin osa oli myönteisiä: erityisesti kysymykset saivat opiskelijat pysymään hereillä ja keskittyneinä, ja kysymyksiä seurannut oikean vastauksen analyysi koettiin opettavaiseksi. Osa ei kuitenkaan kokenut saavansa kysymyksistä mitään irti, ja niiden pohtimiseen käytettiin heidän mielestään liian paljon aikaa.

Emme suinkaan ole vielä luentoäänestysten asiantuntijoita ja aina välillä jopa epäilemme niiden mielekkyyttä. Epäröintiä on lisännyt se, että opiskelijoiden vastausaktiivisuus vähentyy melko radikaalisti kurssin edetessä. Osittain kyse on siitä, että tutustuttuaan toisiinsa opiskelijat ryhtyvät äänestämään entistä enemmän ryhmissä, ja siksi annettujen äänten määrä vähenee. Toisaalta asiaan tuntuu liittyvän uutuudenviehätyksen katoaminen, jonka jälkeen äänestyksestä ei enää innostuta.

Mielestämme opiskelijoiden aktivointi ja omaan ajatteluun kannustaminen on luennolla joka tapauksessa tärkeää, ja sähköiset äänestykset tarjoavat siihen yhden kätevän keinon.

“Aktivointikysymykset pitivät luennolla hereillä. Jos kysymyksiä ei esitetä, luennolla vaipuu koomaan. Näin käy joka kurssilla missä luennolla ei kysytä juuri mitään.”

Tavoitteena oppimisyhteisö

12.9.2013 | Jokke I A Häsä

Kirjoittaneet Jani Hannula, Terhi Hautala, Jokke Häsä ja Johanna Rämö.

Matematiikan ja tilastotieteen laitoksella on jo pitkään työskennelty opiskelijoiden viihtyvyyden lisäämiseksi. Koska matematiikan opiskelu on usein vaativaa, huomiota on kiinnitetty erityisesti siihen, että opiskelijoiden olisi helppo lähestyä henkilökuntaa, kun he kokevat tarvitsevansa apua. Tähän tarpeeseen vastattiin noin kymmenen vuotta sitten ottamalla käyttöön niin kutsuttu laskupaja, jossa opiskelijat saattoivat tehdä yhdessä kotitehtäviä omaan tahtiin. Idea lainattiin Oulun yliopistosta. Paikalla oli ohjaaja, jolta oli mahdollista kysyä neuvoa missä tahansa peruskursseihin liittyvässä ongelmassa.

Laskupajan syntyajoista on opiskeluympäristön kehittämisessä kuljettu jo pitkä matka. Laitoksella on entistä enemmän pyritty lisäämään sekä opiskelijoiden ja opettajien välistä että myös opiskelijoiden keskinäistä vuorovaikutusta. Toisin sanoen opiskelijoita on kannustettu opiskelemaan ja ottamaan asioista selvää yhdessä. Tässä ovat olleet suurena apuna eräät tilankäyttöön liittyvät innovaatiot, joista kerrotaan tarkemmin alla.

Oppimisyhteisön rakentamisessa olennaista on, että opiskelijat saadaan viihtymään laitoksella myös luentojen ja harjoitusten ulkopuolella ja että heidät saadaan keskustelemaan ja työskentelemään yhdessä opintojensa eteen. Kun laitos muutti uusiin tiloihin, varattiin pääkäytävältä keskeltä laitosta tilaa pöydille, joiden ääressä opiskelijat voivat työskennellä. Nämä pöydät järjestettiin ryhmiksi, jotta ne tukisivat opiskelijoiden keskinäistä vuorovaikutusta ja lisäisivät yhteistyötä.

Jo pöydät sinänsä vetivät opiskelijoita puoleensa, luultavasti muun muassa siksi, että ne sijaitsevat opiskelijahuoneen ja monien luokkatilojen välittömässä läheisyydessä. Niiden ääressä työskentelyä on sittemmin helpotettu entisestään lisäämällä käytävän seinille liitutauluja ja päällystämällä pöydät erityisillä plekseillä, jotka tekevät niistä käytännössä tussitauluja. (Pleksilevyt toimitti Merocap oy.)

Mitä sitten tapahtui laskupajalle? Käytävällä olevat pöydät osoittautuivat niin suosituksi opiskelupaikaksi, että erillisestä laskupajaluokasta luovuttiin kokonaan ja laskupajaohjaajat siirtyivät kiertelemään käytävälle opiskelijoiden sekaan. Heidät puettiin keltaisiin huomioliiveihin, jotta he erottuisivat selvästi muista ohikulkijoista.

Fyysisen työympäristön kehittämisen lisäksi laitoksella mietitään edelleen myös muita keinoja, joilla opiskelijoiden kynnystä avun pyytämiseen voisi madaltaa. Viime aikoina on panostettu muun muassa ohjaajien valintaan ja koulutukseen. Kaikki ohjaajat eivät kuulu laitoksen vakinaiseen henkilökuntaan, vaan osa heistä valitaan vanhempien opiskelijoiden joukosta hakemusten ja haastattelujen perusteella. Lisäksi ohjaajat saavat käytännön opastusta siihen, miten opiskelijoita ja heidän ongelmiaan kannattaa lähestyä.

Opiskelijoiden lisäksi myös henkilökunta on ottanut käytävän käyttöönsä. Laitoksen tutkijat pysähtyvät käytävää pitkin kulkiessaan juttelemaan opiskelijoiden tai toisten tutkijoiden kanssa. Jotkut heistä tapaavat käytävällä jatko-opiskelijoitaan ja innostuvat selvittämään asioita liitutaulujen ja pleksipöytien ääressä.

Yllä mainitut järjestelyt näyttävät tuottaneen tulosta. Laitokselle tulija törmää välittömästi opiskelijoihin, jotka kuhisevat liitutaulujen edessä, kirjoittelevat pöytiin kaavoja ja keskustelevat sekä opettajien että toistensa kanssa matematiikasta ja sen opiskelusta. Sivullisetkin näyttävät huomanneen laitoksellamme vallitsevan hyvän opiskeluhengen, ja jopa Ylioppilaslehden päätoimittaja kirjoitti aiheesta tänä keväänä pääkirjoituksessaan.

Kumpula opettaa

Ajatuksia matematiikan opettamisesta

Author Archives: Jokke I A Häsä